Here's a complex ACT geometry question that stops students in their tracks — see if you can solve it

Remember those standardized test questions that always used to stump you?

Well, they still exist.

And they're still hard to solve, even if you're an adult now and have successfully taken the SAT and/or ACT once or even a few times.

We spoke with Tom Rose, the co-founder of Testive, which offers SAT and ACT learning software as well as coaching, who presented us with a particularly tricky problem that stumps students regularly.

Luckily, he also walked us through the difficult geometry question.

Take a look:

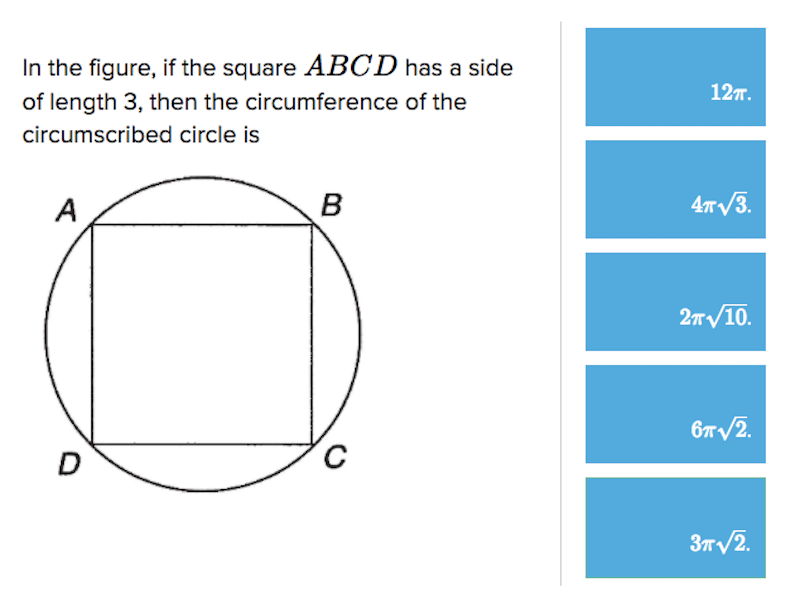Testive

Don't know where to start? Here are the steps Rose suggests taking to solve it:

In order to figure out the circumference of the circle, Rose says we need to first find its diameter, because circumference (c) is equal to pi (π) times diameter (d). In other words: c = πd.

A circle's diameter is the length of a line that goes through its center and touches two points along its edge. So Rose starts off by drawing a line that represents the circle's diameter:

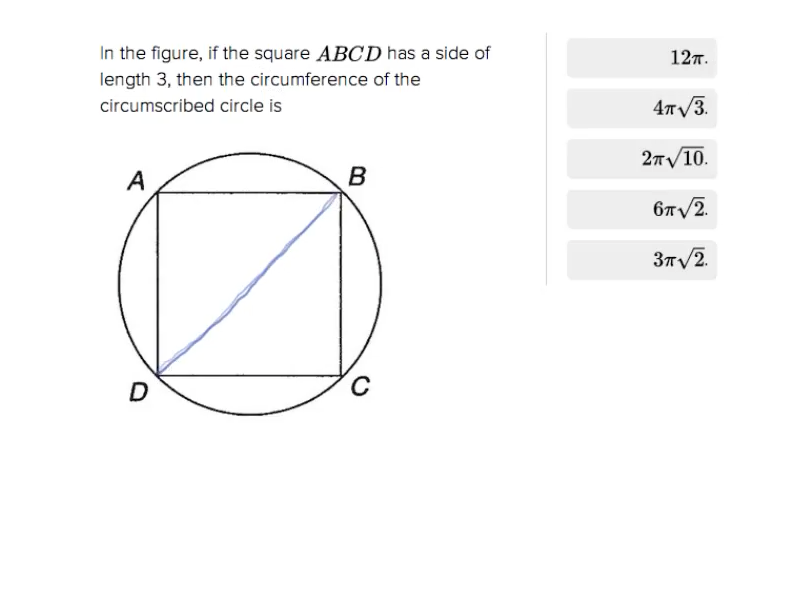Testive

Rose then points out that the triangle he has just created by drawing the line that represents the diameter is an isosceles triangle. He knows it's an isosceles triangle because this kind of triangle must have at least two sides that are the same length.

The question tells us that the square ABCD has a side length of 3, which means all sides have a length of 3 since a square is made up of 4 equal sides.

Squares also have four 90 degree angles, which means that the angle at point C is 90 degrees. Rose then says that the triangle's two other angles must be 45 degrees because all the interior angles of a triangle must add up to 180 (45+45+90 = 180).

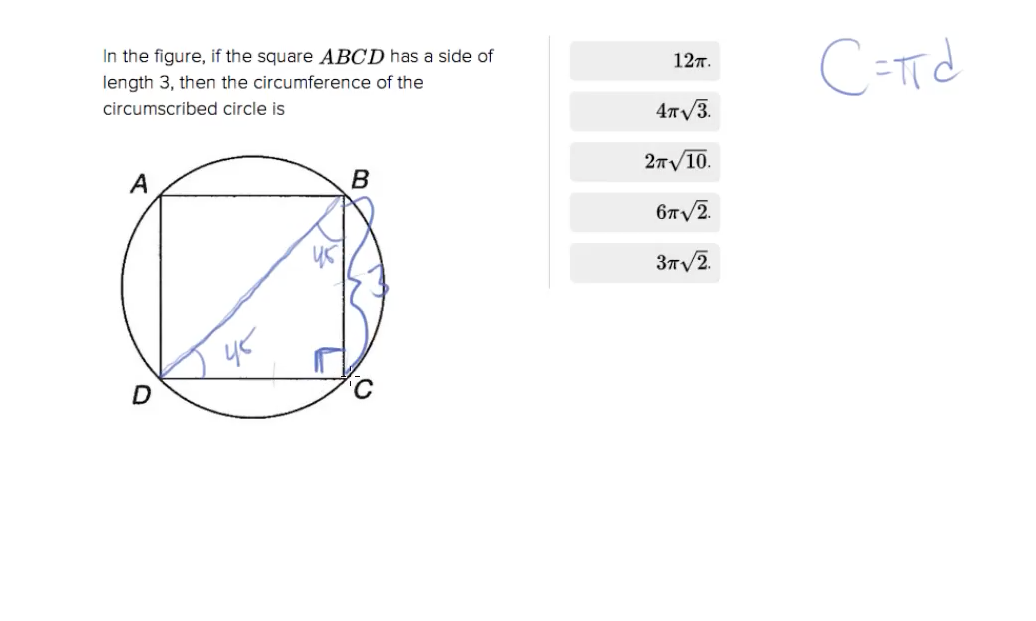Testive

According to Rose, 45, 45, 90 triangles have a special ratio of side lengths that will always be the same (and that students should therefore have memorized). That ratio is 1 to the square root of 2 (√2).  And because we know that two of the triangle's sides are equal to 3, that means the third side has to be equal to 3 times √2 (3√2).

Remember that the third side — which is equal to 3√2 — is the circle's diameter. We're trying to find circumference, which, as stated earlier, is equal to pi times diameter (c = πd). We know that d is equal to 3√2, so the circumference is equal to pi times 3√2 or 3π√2, the last answer of the five listed.

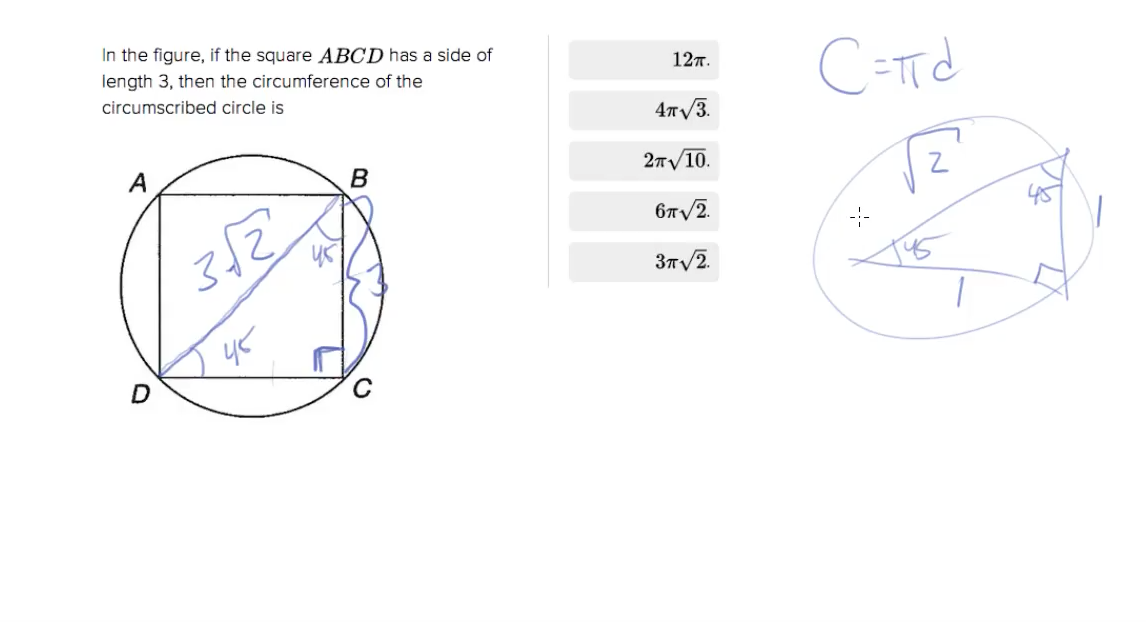 Testive

If you were able to figure that out on your own, kudos to you for paying attention in your high school math class and actually retaining that information.

This isn't the only way to solve this problem, though. For the full explanation and another method, click here.